There has been very little work done in international football analytics compared to the club game . The general consensus is that working with these statistics is much more difficult for a variety of reasons, the most often citied are a small sample size, high turnover of squad composition and varying strengths of schedule.
Looking at the World Cup in isolation the problem of squad turnover is handled as countries are not allowed to change their squad composition once the tournament has begun. Further restricting analysis to the group stage also deals with the strength of schedule problem. Looking solely at the World Cup group stage the only problem becomes the question of sample size.
Leading up to every World Cup journalists may not explicitly use the term sample size, but they all discuss this idea of “getting unlucky” or not “getting the bounces” in the short time frame. This line of inquiry brings up the question, do the best teams really get through the group stage? Or at the very least do the teams that play the best in the opening three matches make it through?
The first question is very difficult to answer for many of the reasons stated above. Going into the World Cup we really don't know who the best teams are. Club form doesn't always translate into national team form and it's difficult to compare underlying talent between teams that have faced very different competition leading up to the tournament. The second question is much easier to deal with, do the teams that play the best in the opening three matches make it through their group?
In order to take a more in-depth look at this question I've chosen simple proxies for playing well and for getting lucky. FIFA does not provide any shot location data, at least none that I've been able to find, so the best available alternative to use for dominance is total shot ratio or TSR. The idea is that over three matches the extent to which a team outshoots their opponents indicates how well they have played. On the flip side I've used PDO as a proxy for luck. These two proxies have often been used elsewhere on Statsbomb.
The data I'm using for this analysis are from the 2010 World Cup. If anyone needs a refresher as to where teams finished in the group stage the final results are all here.
Most teams go into the group stage with the goal of qualifying for the round of sixteen, and the question of getting unlucky only comes up if a team doesn't make it past the group stage, so instead of looking at final position I just look at whether or not the team qualified for the next round.
I use a probit model, which assigns a probability to each team getting out of the group stage given their TSR and PDO throughout the three group stage matches. Each team assigned a probability greater than 0.5 is expected to qualify and each team with a probability less than 0.5 is expected to be eliminated.
The first thing we want to understand is how many of these teams the model accurately assigned a probability of greater than 0.5. Or in other words how many of the teams fates can be described purely using these two statistics.
The complete model accurately anticipates fourteen of the sixteen teams that made the second round. The only two teams that the model did not accurately assign to the round of sixteen were South Korea and Slovakia.
Essentially this means that neither the proxy for skill nor the proxy for luck can explain why these two teams qualified for the round of sixteen. Examining these two teams in context gives a bit more insight. South Korea's below average TSR seems to be down to the curse of the small sample size in which they are disproportionately punished for a 4-1 pummelling by Argentina. As for Slovakia they seem to just have been even luckier to escape a group with Paraguay and Italy than their 1115 PDO indicates.
Now we use two restricted models one which only uses PDO and one which only uses TSR. The model only taking into account PDO correctly predicts eleven of the teams that qualified, whereas the model only taking TSR into account correctly predicts thirteen of the teams that qualified. This suggests that despite the small sample size the quality of performance has a bigger impact on whether or not a team qualifies than luck does.
This becomes more interesting when comparing the teams that the PDO and TSR models differ on. The PDO model correctly predicted two teams to qualify that the TSR model didn't: Slovakia and Mexico. As mentioned above Slovakia is a bit of an outlier since even the combined model didn't anticipate their PDO to be high enough to make up for their poor TSR.
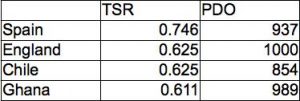
The TSR model correctly predicted four teams to qualify that the PDO model didn't, including the tournament champions Spain.
It is interesting that all of these four teams which made up for their relative “unluckiness” did so with TSRs greater than 0.6. The most telling number here might be Chile's who appear to have been very unlucky with a PDO of 854, but were able to overcome it by significantly outshooting their opponents.
The evidence from the 2010 World Cup suggests that if a team outshoots their opponents throughout the group stage by at least 6 to 4 they should be able to get past so called “unlucky bounces”. The data also show that getting lucky in the group stage and making it to the round of sixteen is not impossible, but it doesn't appear to be quite as prominent as many TV commentators will inevitably claim during this summer's World Cup.